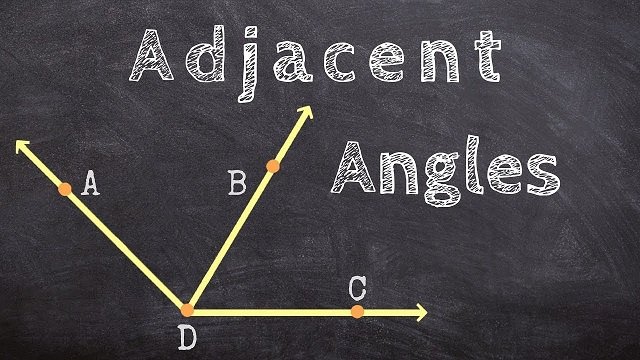
Understanding the concept of adjacent angles is a fundamental part of learning geometry. Adjacent angles are two angles that share a common side and a common vertex but do not overlap. In this article, we will break down the concept of adjacent angles and provide step-by-step examples to help students grasp this important geometric principle Adjacent Angles Examples.
What Are Adjacent Angles?
Adjacent angles are pairs of angles that have the following characteristics:
- Common Vertex: The point where the two angles meet.
- Common Side: A line segment that is a part of both angles.
- Non-Overlapping: The two angles do not cover each other; they lie next to each other.
Understanding these properties is crucial in identifying and working with adjacent angles in various geometric problems Adjacent Angles Examples.
Why Are Adjacent Angles Important?
Adjacent angles are fundamental in geometry as they are often used in proofs and solving problems involving polygons, parallel lines, and transversals. Understanding how to work with adjacent angles can simplify many complex geometric problems Adjacent Angles Examples.
Step-by-Step Examples
Let’s go through a few step-by-step examples to solidify our understanding of adjacent angles.
Example 1: Identifying Adjacent Angles
Problem: Identify the adjacent angles in the following diagram.

Step 1: Locate the Common Vertex In the diagram, point C is a common vertex where multiple angles meet.
Step 2: Identify the Common Side We need to find pairs of angles that share a common side. For instance, angle ∠ACD and angle ∠DCE share the side CD.
Step 3: Ensure the Angles Are Non-Overlapping Ensure that the identified angles do not overlap. In this case, ∠ACD and ∠DCE are next to each other without overlapping Adjacent Angles Examples.
Answer: The adjacent angles in the diagram are ∠ACD and ∠DCE.
Example 2: Calculating Adjacent Angles
Problem: Given that angle ∠ACB is 70 degrees, find the measure of ∠BCD if ∠ACB and ∠BCD are adjacent and form a straight line.
Step 1: Understand the Relationship Since ∠ACB and ∠BCD are adjacent and form a straight line, they are supplementary angles. This means their measures add up to 180 degrees.
Step 2: Set Up the Equation Let ∠BCD be represented by x. Therefore, we have: ∠ACB + ∠BCD = 180 degrees 70 degrees + x = 180 degrees
Step 3: Solve for x Subtract 70 degrees from both sides: x = 180 degrees – 70 degrees x = 110 degrees
Answer: The measure of ∠BCD is 110 degrees.
Example 3: Adjacent Angles in Polygons
Problem: In a quadrilateral, one of the interior angles is 90 degrees, and the adjacent angle is twice the measure of the 90-degree angle. Find the measure of the adjacent angle.
Step 1: Identify the Given Information We are given that one angle is 90 degrees and the adjacent angle is twice this measure.
Step 2: Set Up the Relationship Let the measure of the adjacent angle be x. According to the problem, x = 2 * 90 degrees.
Step 3: Calculate the Adjacent Angle x = 2 * 90 degrees x = 180 degrees
Answer: The measure of the adjacent angle is 180 degrees.
Practice Problems
To further solidify your understanding, try solving these practice problems on your own:
- Problem 1: In the following diagram, identify the pairs of adjacent angles.

- Problem 2: If ∠GHJ is 45 degrees and ∠JHI are adjacent and form a straight line, find the measure of ∠JHI.
- Problem 3: In a pentagon, one of the interior angles is 120 degrees, and the adjacent angle is 60 degrees more than the 120-degree angle. Find the measure of the Adjacent Angles Examples .
Conclusion
Adjacent angles are a fundamental concept in geometry that helps students understand more complex geometric relationships and properties. By identifying the common vertex and side and ensuring the angles do not overlap, students can easily identify and work with adjacent angles. Practice these concepts with various diagrams and problems to master the identification and calculation of Adjacent Angles Examples.